Quite simply, there are things that alter population densities that are a function of the population's density and things that have an effect regardless of the population's density. These are what scientists mean by density-dependence and density-independence. Density-dependent factors tend to be biological interactions - predation, competition, disease. Density-independent factors tend to be catastrophic events - floods, manure spills resulting in fish kills, or a late spring or early fall frost that kills vegetation or insects.

First it is important to understand that what we are interested in with populations is their density (number per area or volume) more so than we are interested in their absolute numbers much of the time. Biological rates - such as birth and death and immigration and emigration rates are often functions of a populations density. Birth rates often decrease as population density increases and death rates tend to increase as density increases. Similarly, more individuals leave the population - immigrate - and fewer enter the population - emigrate - when densities are high. Most of the time, high and low densities are in reference to the population's carrying capacity (K - more on this later) or the maximum number of individuals that an area can support.

Population Growth
You probably remember exponential and logistic growth from school or maybe you have put that all behind you, or so you think. Maybe you remember them as "J-curves" (exponential) and "S-curves" (logistic). Or remember talking about compound interests - a concept similar to exponential population growth.
We sometimes think of populations as growing exponentially - without limits - without the effects of density. Exponential growth is based on the same math as compound interest. Often instead of a true exponential model where populations (or money), is compounded "instantaneously", we have a geometric model which is applied to organisms like deer, most plants, and trout that reproduce and are born at discrete times each year. In geometric growth, we have annual rates of growth, not the instantaneous rates of the exponential model. Trout populations will never be higher than they are when year's eggs hatch into fry. In either case, exponential and geometric (or often times referred to as a discrete exponential model) grow at the same rate over time, without limit.
Of course, it does not take a population ecologist to figure out that no population can grow at the same rate without limit. Or as we talk about it as biologists, there is density-dependence at some time and place. All population have to be regulated by density - they can't grow forever without limit. We may never see the effect of density in their population dynamics (changes over time) because their population never approaches carrying capacity. This tends to be pretty rare in trout streams - most trout populations and density-dependently controlled.
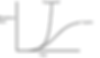
Image Source: Study Blue - Wildlife and Fisheries Sciences
Density-Dependence
Density-dependent population regulation occurs when the demographic rates - those birth, death, immigration, and emigration rates mentioned earlier - change as a function of the population's density. Inverse density dependent factors are those that decrease as the population density increases. Typically, births and immigration are inverse density dependent factors whereas deaths and emigrants are directly related to the population density - they increase as the density increases.

Image from: Chegg Prep - Population Ecology
The next idea to understand is carrying capacity, or "K". The idea behind carrying capacity is it is the maximum population that can be sustained in an area. Once a population reaching K, it theoretically stays there forever and ever. But of course nothing is that simple.
Carrying capacity is set by biotic interactions, in particular competition but also predation, parasitism, and other density-dependent factors set K. Competition is the simplest factor to think about. As a population density approaches K, there are fewer resources available. This means that as a population grows closer to K, competition is more important and individuals in the population may have fewer offspring (reducing the population birth rate) and be less likely to survive (increasing the population death rate). Similarly, disease spreads faster when the population is more dense. Predation often increases as the density increases as well. Think about your favorite hatch, the first 15 minutes may see mayflies largely uneaten but after grabbing the trouts' attention, there are rising fishes everywhere. Trout have responded to the density of the mayflies. For the mayflies, their mortality rates have increased as a function of their density.

Of course, carrying capacity can't be this simple. We might see streams with the same biomass (measurement of weight of the population) but very different populations. You can have a stream with 500 Brown trout that average 2 pounds or 2,000 Brown trout that average half a pound. The biomass is the same, the population is much different. Carrying capacity also changes year to year. We tend to treat K as a constant but it ebbs and flows as the environment changes. If the spaces between rocks fill with sediment and there is less clean spawning gravel, K will decrease because trout will spawn less successfully, lowering their birth rate.
Density-Independence
All populations are subject to density-independent factors. Catastrophic events that alter their populations independent of their density occur generally at random. For streams, the classic example are flood events. Floods reduce populations regardless of the population's density. When a flood occurs, it kills some random proportion of the population. Or a manure spill that results in a fish kill - it would kill 27% (a number I made up) if the density was 1,000 fish per mile or 2,000 fish per mile. Density-independent factors are mostly random - they occur or don't occur randomly and the magnitude of their effect is unpredictable.
Putting it all Together...
When we look at real life examples, they're more interesting and complex than the simple examples. Density-dependence (D-D - mostly because I've gotten tired of writing it out each time) factors and density-independent (D-I) events are each occurring and altering populations. They interact and D-I events can have D-D effects over time.

Figure 3 shows mortality events - a drought, a severe winter, a hurricane, and a fire - that all have different effects on a population. Hurricanes and fires are pretty straight-forward. They are D-I events whose effects on the population are random both in terms of timing and magnitude. We might thing of drought as D-I, it is a random event, but if it continues over time, it may be D-D because it reduces K as there is less space, less food, it concentrates the population increasing predation.

Let us imagine that we have a flood - the classic example of a D-I event. We don't really have to imagine, we have had no shortage of these lately. Just about everywhere in the US is familiar with floods over the past couple of decades. Floods are classic D-I events - they have a mortality effect on the population unrelated to the population's density. Their effect is greater in the spring just after trout emergence or in the winter before emergence (they scour the bottom, digging up trout redds) and lower in the late summer and early fall when trout are in prime condition and young-of-the-year are better able to withstand floods.

There are also D-D effects of a flood event such as the 2018 flood we experienced (and the 2019 flood). Floods had effects that increased birth rates - there is more clean spawning gravel than ever. But floods also had a inverse D-D effect by reducing grasshopper and other terrestrial insect populations which are critical for late summer and early fall trout (see Aquatic Insects in the Spring, Terrestrials in the Fall for more details about reciprocal prey subsidies). They've created better habitat, increasing K, or they could have "blown out" habitat features, reducing K. The point is, floods are D-I events but there are also effects of floods that are D-D.
To sum it up, populations are regulated by a complex interaction of regulating factors, changing carrying capacities, and we have barely touched upon the effects of humans. I can't - and you don't want me to - try to do it all in a single post.